ELECTROCHEMISTRY

Electrochemistry

Electrons flowing from one end to another generates a current. Chemical reactions that involve redox reactions can be separated into containers called electrodes. The solution of charged particle is usually called an electrolyte. Negative charged particles are called Anions, and the positive charged particles are called Cations. Moreover, electron flow from one electrode to the other electrode be measured by using a voltmeter. Electrodes have charges due to electron flow.

The negative electrode is called a cathode since they receive cations. However, the positive electrode is called an anode since they receive anions. This helps charges to balance out in the whole system while generating electricity. Sometimes there is a bridge of salt connected to across the system to balance out the charges. This is called the Salt bridge. The amount of electricity produced can be calculated from the moles of substance used up or produced from the electrochemical reaction.

Electrochemical / Activity series

A + B ^________> A ⁺ + B^–      , this means element A has given an electron to element B to become A + and B- . A has reduced B . Therefore, A is reducing agent [it is oxidized to form product A+]. On the other hand, B has taken an electron from A. this means B oxidized A . Therefore, B is oxidizing agent [ it is reduced to form product B-]  .   

Transfer of electrons from a place of higher oxidation potential to a place of lower oxidation potential creates a potential difference. In other words, electrons flow from a lower reduction potential to a higher reduction potential. The flow of electrons in one direction is a force [aka electromotive force ( e )  = emf ] that creates electrical energy  or electricity that can be measured.

The power of an element to reduce or give electrons to Hydrogens ions to form hydrogen gas and become OXIDIZED at 25^oC is called standard oxidation potential.

Positive values mean reaction is feasible in forward direction as written.

E.g. 2A + 2H^{+ _________}>  2A ⁺ + H₂   (gas)

Negative value means reaction will occur in reverse direction .

2A ⁺ + H₂   (gas) ^_________>  2A + 2H⁺

Electrochemical / activity series [ability of an element to be oxidized by hydrogen ions]

2K + 2H^{+ ___________}> 2K⁺ + H_{2 (g)}…………2.93V

2Al + 6H^{+ ___________}> 2Al³⁺ + 3H_{2 (g)}……..1.66V

Zn + 2H^{+ ___________}> Zn²⁺ + H_{2 (g)}…………0.76V

2Pb + 2H^{+ ___________}> 2Pb⁺ + H_{2 (g)}………..0.13V

e- + 2H^{+ ___________}>  H_{2 (g)}  [standard]…… .0.00V

2Cu + 2H^{+ ___________}> Cu²⁺ + H_{2 (g)}………-0.34V

2I- + 2H^{+ ___________}> I₂ + H_{2 (g)}……………-0.53V

2Fe²⁺ + 2H^{+ ___________}> 2Fe³⁺ + H_{2 (g)}……..-0.77V

2F- + 2H^{+ ___________}> F₂     +    H_{2 (g)}………-2.87V

The element of higher potential can reduce an element of lower potential for a chemical reaction to occur in the direction shown.

E.g. 2Mg + O₂ ^_______> 2MgO [ reaction occur in forward direction because of huge difference in oxidation potential of magnesium and oxygen]

Use a table of electrochemical/activity series to predict if the following reactions will occur as written?

K⁺ + Li ^________> K + Li⁺

K + Li^+________> K⁺ + Li

KCl + Li ^______> K + LiCl

Sn²⁺  + Cu ^{2+ ________}> Sn⁴⁺  + Cu

Sn⁴⁺  + Cu ^________> Sn²⁺  + Cu ²⁺

SnO₂ + Cu ^_____> SnO  + CuO

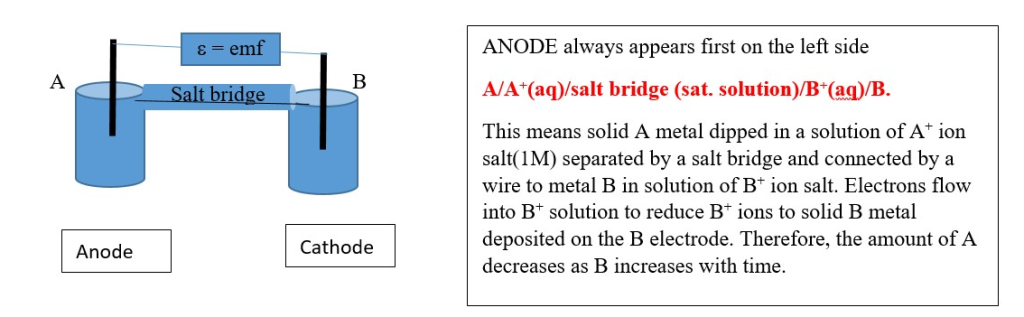
ELECTROCHEMICAL CELL DIAGRAM AND NOTATION

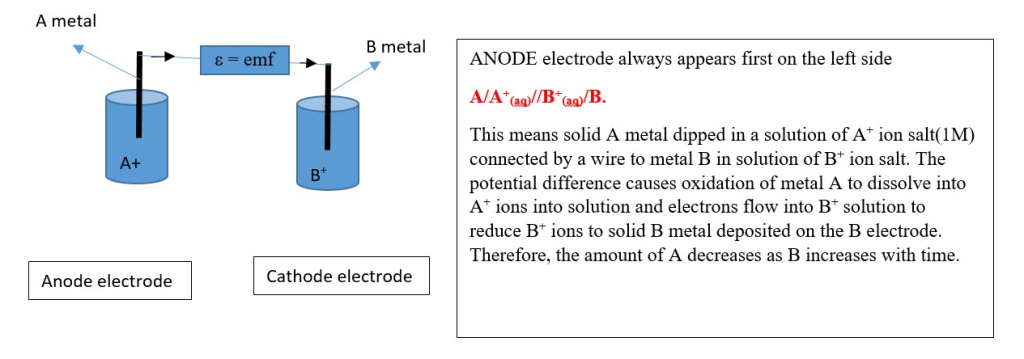
Junction potentials will decrease the overall emf. A salt bridge can be introduced to minimize junction potentials in both electrodes.

Half cell reactions

ANODE:           [oxidation]      A ^_________> A ⁺  + e-  ……….emf = X

CATHODE:      [ reduction]     B ⁺  + e- ^________> B ………..emf = Y

Overall reaction: A  + B^{+ ________}> A⁺   +    B ……. Cell emf = emf[anode] + emf[cathode] = X + Y

Cell emf  = [RT/nF]Ink.

              =[0.059V/n] logK

NOTE : The stoichiometry does not affect the value of the electric potential difference.

e.g Electrochemical cell made of aluminum anode and hydrogen cathode [hydrogen with  Pt inert metal].

The Pt does not affect the cell potential but allows electrons to flow into solution to reduce H+ IONS to H₂ gas.

[Al/Al(NO₃)₃(1.0M)//HNO₃(1.0M)/H₂/Pt]

Anode reaction: Al + Al(NO₃)₃ (aq) ^______> Al³⁺+ 3NO₃^–    + Al³⁺+3e-

We don’t have to write all this but just show the species oxidized at the anode and forget about inert counter ions. Solid Al is oxidized to aqueous Al₃⁺ ions.

Al ^_____> Al ³⁺+ 3e-…………1.66V

Cathode: H⁺ + NO₃ + e-  ^______> H₂(g) + NO₃^–

We don’t have to write all this but just show species reduced (H⁺) and forget about counter ion NO₃^–

H⁺ (aq) + e-  ^______> H₂(g) ……0.00V

[need to multiply left by 2 to balance H but multiplying does not affect the reduction or oxidation potential.]

2H⁺ (aq) + 2e-  ^______> H₂(g) ……0.00V [DO NOT MULTIPLY]

Al ^_____> Al ³⁺+ 3e-…………1.66V ………..equation1

2H⁺ (aq) + 2e-  ^______> H₂(g) ……0.00V……equation 2

Multiply equation 1 by 2 and equation 2 by 3 to balance the number of electrons in the anode and cathode reactions.

2Al ^_____> 2Al ³⁺+ 6e-…………1.66V [do not multiply]………..new equation 1

6H⁺ (aq) + 6e-  ^______> 3H₂(g) ……0.00V……………………….new equation 2

Add to get overall reaction:

2Al(s) + 6H⁺ (aq)  ^_____> 2Al ³⁺   + 3H₂(g)

Overall emf = 1.66 + 0.00 = 1.66V

NOTE: Using oxidation potential values we add the potential values [emf =Anode + Cathode].

However, if your table in your textbook is in reduction potential values and you don’t want to change the sign of the anode potential, then you have to subtract ANODE potential from CATHODE potential, [emf= Cathode – Anode]

Reaction equilibria and emf measurement.

G = G^o  +  RTInQ     ,   where Q is reaction quotient

ΔG = ΔG^o  +  RTInQ    .

-nFemf = -nFemf^o  +  RTInQ    .

emf=emf^o – [RT/nF]InQ.

      =emf^o – 0.059V/n[logQ] …. Nernst equation.

K or Q can be ion concentration [in molarity] or gas pressure [in atm or bar ]. Solids are omitted.

K or Q = [product]/[reactants]

e.g. A(s) + B(s)  +H₂O ^_________> A⁺ (aq) +  B^–(aq)

emf = emf^o – 0.059V/n[log[A⁺][B^–]………

n is number of electrons involved in the balanced chemical reaction. Solid A and B are omitted in the Nerst equation.

Therefore, emf=emf^o – 0.059V/[log[A⁺][B^–],

Standard reduction potentials or standard oxidation potentials (emf^o) are given in tables in your textbook. The values are the same but opposite in sign.

For the cell reaction, A(s) + 2B⁺(aq)  ^_____> B₂(gas) + A⁺²(aq)

Cell notation is A/A⁺²/B⁺/B₂

emf=emf^o – 0.059V/2[log([A⁺] (P_B2)/[B⁺]²)] , value of n = 2

where P_B2   is the pressure of gas B_{2 .}

Example :

Write the expression of the emf (e) under different conditions other than 1atm and 1.0M concentrations for the example above.

2Al(s) + 6H⁺ (aq)  ^_____> 2Al ³⁺   + 3H₂(g)

emf = emf^o – [RT/nF]InQ ,

where n=6 and Q=[product]/[reactants]

emf = emf^o – [RT/nF]In[([2Al ³⁺ ]² P_H2³ ) / ([H⁺]⁶[Al])],

Al solid is omitted since activity or concentration is taken as 1.

emf = emf^o – [RT/6F]In[([Al ³⁺ ]² P_H2³ ) / ([H⁺]⁶)]

emf = 1.66 – [RT/6F]In[([Al ³⁺ ]² P_H2³ ) / ([H⁺]⁶)]

Calculate the pH of the nitric acid if the cell emf is 1.02V and the concentration of Al(NO₃)₃ is 1.89M and the pressure of the gas is 2.5 atm.

emf = 1.66 – [RT/6F]In[([Al ³⁺ ]² P_H2³ ) / ([H⁺]⁶)]

1.02V =1.66V – (0.059V/6) x [log([([Al ³⁺ ]² P_H2³ ) / ([H⁺]⁶)])  

1.02V =1.66V – (0.059V/6) x [log([([1.89M ]²  X (2.5atm )³ / ([H⁺]⁶)])  

(0.059V/6) x  [log([([1.89M ]²  X (2.5atm)³ / ([H⁺]⁶)])  = 1.66V – 1.02V = 0.64V

[log([([1.89M ]²  X (2.5atm )³ / ([H⁺]⁶)])  = 0.64V x 6 / 0.059V = 65.085

The values in the brackets are already divided by standard units so no units in brackets.

log([([1.89 ]²  X (2.5 )³ / ([H⁺]⁶)])  =  65.085

([([1.89 ]²  X (2.5 )³ / ([H⁺]⁶)])   = e^{(65.085 x 2.303)}   = 1.25 x 10⁶⁵

([H⁺]⁶)])   = [1.89 ]²  X (2.5 )³   /  1.25 x 10⁶⁵

                 = 0.000028028

[H⁺]   = (0.000028028)^1/6

               =0.1743

pH = -log[H⁺]   .

     = -log[0.1743]  = 0.76

EQUILIBRIUM SITUATION

Equilibrium means K = Q and ΔG = 0

0 = ΔG^o  +  RTInQ 

 therefore, we can deduce that  0 = emf^o – [RT/nF]InQ

emf^o = [RT/nF]InQ ,   or     emf^o  = 0.059V/n[logQ]

from this we can calculate the equilibrium constant since Q=K and then estimate the minimum amount of substance needed to shift equilibrium forward or backwards.